Richard M. Christensen |
||
|
||||
Recent Additons |
||||||
Key Junctures |
||
 |
||||
General Matters |
||||
 |
||||
 |
||||||||
 |
||||||||
 |
||||||||
 |
||||||||
Can Atomic/Nano Scale |
||||||||
 |
||||||
 |
||||||
 |
||||||
 |
||||||
 |
||||||||
 |
||||||||
 |
||||||
 |
||||||
 |
||||||||
 |
||||||||
 |
||||||
Copyright© 2019 |
||||||
The World Wide Failure Exercise II
Examination of Results
 The final results have been given for an active and long lived evaluation program of the failure theories for fiber composite materials. The entire process of the WWFE-II extended over a period of many years. This “failure exercise” was conducted by Hinton and Kaddour and the final summing up of results is given in Kaddour and Hinton [1]. Since this project was a large scope, high exposure, long term effort on failure criteria, its completion should be noted here and its contents surveyed. Accordingly, the present account involves a critical and thorough examination of the results and conclusions formed in [1]. This appraisal of [1] is organized such that it can easily be read and understood without side by side, immediate reference to [1]. Ref. [1] can be consulted either prior to or following the reading of this review.
The final results have been given for an active and long lived evaluation program of the failure theories for fiber composite materials. The entire process of the WWFE-II extended over a period of many years. This “failure exercise” was conducted by Hinton and Kaddour and the final summing up of results is given in Kaddour and Hinton [1]. Since this project was a large scope, high exposure, long term effort on failure criteria, its completion should be noted here and its contents surveyed. Accordingly, the present account involves a critical and thorough examination of the results and conclusions formed in [1]. This appraisal of [1] is organized such that it can easily be read and understood without side by side, immediate reference to [1]. Ref. [1] can be consulted either prior to or following the reading of this review.
Failure Exercise Setup
The large majority of the comparisons between the theories of failure and the data sets were for the failure characteristics of unidirectional laminas and some specific laminates. Both carbon and glass fiber types were involved, all with polymeric matrix phases. Only one data set concerned a homogeneous polymeric resin.
There were 12 different failure theories for fiber composites under consideration. All but 2 of these involved the originators of their particular theory in the process of comparing theoretical predictions with data supplied by the originators of the study. Two of the 12 theories were those of Hashin and of Christensen, both of whom declined to participate in the failure exercise. Others apparently were assigned to represent those two theories. The terms “authors” will be used in connection with the associated papers written on behalf of each individual theory. The term “organizers” will be used in designation of the organizers of the failure exercise, even though they also authored the final exposition of results, [1], as well as many previous pieces in the overall program.
The failure exercise occurred in two stages. First a blind comparison with data occurred for specified stress states. That was followed by revisions to the theories made by the authors. It is this second round of results that will be of concern here since presumably it represents the best performance by each associated theory. These revised theories were designated by the suffix “B”, e.g. “Smith B” by the organizers in the results in [1].
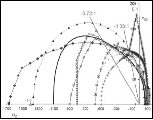
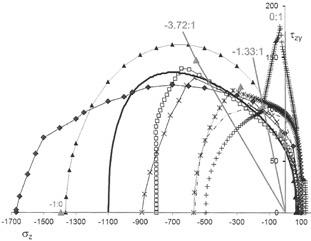
The final results were stated by rank order lists of the theories, each with associated grades of performance. Actually, there were two lists, first was the rank order list of “quantitative assessments” which were based explicitly and solely on comparisons with data: Fig. 25, page 960 of [1]. Second was a rank order list of “quantitative and qualitative assessments”, into which was factored the organizers view of the quality of the respective theories: Fig. 26, page 960 of [1]. The latter rank orderings will herein be referred to as the quant. & qual. rankings.
Before getting into the substance and details of the failure exercise, it should be noted that all 12 of the investigations were serious, thoughtful efforts. There were no quick or casual passes at the problem. It is obvious that everyone was committed to making improvements in the field. But the tremendous scatter and divergence of results between the 12 different approaches provides stark testimony to the difficulty of the task of modeling failure. Nevertheless and regardless of the outcome, the 12 author sets and the organizers as well have commendably extended themselves and deserve recognition for their efforts on this extraordinarily difficult problem.
The Philosophical Challenge
When one looks at the quantitative rankings (comparisons with data) given in Fig. 25, [1], the first impression is that for all 12 theories there is not much difference between adjacent entries in the rank ordering list, just a gradual gradation from one to the next and the next and so on. No theory/model scored perfect agreement with the data. There was no single model that stood out while all others were mediocre or poor. Every model had hits and misses, some a little more than others but all with a very wide distribution of results. From just this much information, the preliminary conclusion would seem to be that there was no conclusion, no meaningful conclusion. There certainly was no single failure criterion to be recommended for use while all others would be rejected. This much is purely concerned with the quantitative results. The qualitative assessments will be considered a little later in this examination.
Now to get into the specifics, it quickly becomes even more open ended and uncertain. There is one issue that dominates and supersedes all others. After discussing a wide variety of details the organizers state on page 965 of [1] that “one of the major philosophical points to emerge from WWFE-II is the diversity exhibited between the theories as to whether certain failure envelopes are open or closed.” This refers to lamina and laminate predictions under either 3-D hydrostatic pressure or under transverse 2-D hydrostatic pressure for lamina. Six of the 12 failure theories predicted open failure envelopes behavior in some of the cases, implying some infinite stress components without failure. The other 6 theories did not predict open failure envelopes, thus had no unbounded failure stresses. This crucial difference should have provided a critical and decisive fulcrum from which to better judge the results. Unfortunately that was not done. Instead, the final sentence in the final paper of the entire failure exercise [1] concluded by saying “This remains a critical philosophical challenge to future researchers in the field.”
There is no need to wait for the hypothetical future researchers to render a verdict. Some straight line mechanics of materials reasoning provides the answer to the organizer’s basic question. It would be utterly impossible for high stiffness, high strength fiber composite materials to withstand unlimited (or absurdly large) hydrostatic pressure loads without failing. Here’s why.
First consider a fiber/matrix lamina under transverse 2-D hydrostatic pressure. For very high stiffness fibers such as in carbon fiber composites, the deformation state in the polymeric matrix phase is effectively that of plane strain. It is the matrix phase that will failure in these stress states. Idealize the matrix as a homogeneous and isotropic material under plane strain conditions. Work out the failure stresses in this homogeneous matrix material under plane strain for two loading conditions, (i) transverse uniaxial stress and (ii) transverse eqi-biaxial stresses. Reasonable estimates of these failure stress magnitudes can be determined using critical distortional energy. As will be seen, this will be sufficient to show that unlimited distortional stresses in fiber composites cannot be sustained.
This procedure is easily carried out and the results are as follows. For a matrix material Poisson’s ratio of 1/3, the eqi-biaxial compressive stresses at failure are 2.65 times the uniaxial compressive stress at failure. For a matrix material Poisson’s ratio of 0.35, the eqi-biaxial compressive stresses at failure are 2.93 times the uniaxial compressive stress at failure. These ratios are indicative of the corresponding strength ratios for the fiber composite lamina since it is matrix controlled with respect to failure in these stress states. The bottom line is that the transverse hydrostatic pressure at failure is about 2 or 3 or 4 times that of the transverse uniaxial compressive stress at failure. High performance fiber composite materials absolutely cannot support unlimited hydrostatic pressure loads with failing. The distortional state of deformation in the matrix phase is far too great. Isotropic matrix materials cannot withstand unlimited or virtually unlimited distortional stresses. Only if there were no distortional stresses in the matrix phase could this occur.
The above reasoning is for the transverse pressure in a lamina. The same results also apply for the 3-D hydrostatic pressure case because for very high stiffness fibers the fiber phase supports the axial load and the matrix phase still sees an effectively plane strain condition. The compressive strengths under 2-D and 3-D hydrostatic pressures are quite large, meaning that they are at least 2 times or more likely about 3 or 4 times the transverse uniaxial compressive strength, but they still are of the same order, and must be considered in all applications. To model these strengths as being unlimited would be an unacceptable defect in any failure theory.
Now to get back to the results of the failure exercise, still for the quantitative assessments, the top ranked 4 theories (Cuntze, Carrere, Pinho, Wolfe) in Fig. 25 all predicted unlimited or almost unlimited load capability in some of the hydrostatic pressure cases. All 4 of these theories are fundamentally deficient in this most important of all assessments. Not only are these top 4 theories seriously incorrect on this basic issue, they are extremely unconservative as well. This does not mean that these 4 theories cannot be revised and repaired, but it does mean that the rank order lists as given in the failure exercise must be called into question. There is a major disconnect here.
Other Challenges
Several other aspects of the WWFE-II evaluation should be and will also be discussed here since they too carry cautionary notes that are of importance for failure criteria development and failure criteria evaluation.
Perhaps because the quantitative results were not viewed as being decisive enough, the organizers may have felt the need to reinforce the conclusions by including their own views on the validity of each of the 12 theories. They did this by making separate and subjective valuations of the capability of each model to “capture” the trends that they (the organizers) could see in the data cases. They called these the “qualitative assessments” and combined them with the quantitative assessments to form the rank ordered “quantitative and qualitative assessments” as their final recommendation and as shown in Fig. 26, [1]. As already mentioned, this will be referred to as the quant. & qual. assessments.
Also recalling that with the exception of 2 theories that were not revised, those theories in Fig. 26 labeled with the suffix B are those involving revisions and are those considered here, plus the two unrevised theories. Now get down to further specific cases. The particular theory that was ranked as 6th (Christensen) out of the 12 in the quantitative evaluation was lowered to 11th out of 12 in the quant. & qual. evaluation. For this theory to take such a devaluation must mean that it was viewed by the organizers as being of the very poorest of quality.
In contrast, the theory that was nearly at the bottom and ranked as 11th (Puck) out of 12 in the quantitative evaluation was elevated to 7th out of 12 in the quant. & qual. evaluation. The organizers were quite open in saying that “it is the organizers’ view that the theory is based on a solid foundation”. They did not offer any substantive reasons to support this view. Furthermore this model was included with the top ranked 3 models from the quantitative assessment as comprising the overall top 4 theories (Pinho, Carrere, Puck, Cuntze) forming Group 1 where as stated, “Group 1 contains fairly developed models which are judged to be in an advanced stage and near completion.” Group 2 contained all other, and in their view, lessor models needing further work.
So the theory that was nearly at the bottom in the quantitative assessment ended up with a highest 4 overall recommendation. It also is a surprising claim from the organizers that there are multiple (four) failure models that are nearly ready for general application. How could there be 4 different mutually contradictory theories of failure each of which still assures success in applications. There are not 4 different theories of elasticity each of which is equally capable and acceptable.
As the above two examples reveal, it should be recognized that this is not just a rank ordered result from a data generated scoring system, but that the final recommendations contain elements of the judgments and preferences of the organizers. This is perfectly alright so long as it is clearly expressed and well understood, which it is. Of course this then means that this is not an evaluation in the usual strict sense of the term but rather some much broader and looser type of evaluation.
The technical meaning of the term failure criteria was confused with the implications of stress analysis in [1]. Both are required but the two are absolutely independent entities. For example, the organizers cited and criticized some failure criteria results as being linear when it appears that they meant that the stress analyses that were used to implement the failure criteria were linear. The failure criterion that was being referred to was certainly nonlinear. Most or perhaps all of the failure criteria in the failure exercise are nonlinear. It is obvious that some of the stress analyses must be taken to be nonlinear in some cases to determine the stresses and to extract the failure stresses, the state of axial shear being the prime example. Failure to perform a nonlinear stress analysis when needed should not have penalized the associated failure criterion, especially when its originator was not involved in the failure exercise. Matters such as this add further uncertainty and confusion to the comparisons of the different failure criteria.
Although the failure exercise had one data case on an isotropic material (epoxy) there seemed to be a strange lack of interest or any understanding of the framework for the failure of isotropic materials. There was no separate theoretical assessment of the field of isotropic materials failure. It is very hard to conceive of getting fiber composites failure right and correct if first there is not a solid foundation of isotropic materials failure to build upon.
Still on the subject of materials types, there was no mention of the possibility that carbon fiber composites and glass fiber composites may require two distinctly different types of failure treatments. It is a very important matter to be considered, especially since the anisotropy ratios vary so widely between the two types. The topic certainly should fall under the heading of a comprehensive account of fiber composites failure.
General Considerations
A logical sequence and natural progression of failure cases to be studied could be those of:
- Isotropic materials
- Unidirectional fiber composites
- Thin quasi-isotropic fiber composite laminates (in-plane)
- Thick quasi-isotropic fiber composite laminates (in-plane and out-of-plane)
- Other common fiber composites laminates
- Woven, braided, twisted forms etc.
Regarding these steps of advancement, it not only would be good practice, but actually seem to be highly important to not move on to the next level until the failure behavior at the previous level was fully understood and consummated. Such a priority protocol as this was not followed in the failure exercise, everything was indiscriminately mixed together and weighted equally.
It is interesting and relevant that there are at least three distinctly different types of technical approaches given in the failure exercise. These are:
- Failure modes
- Micromechanics
- Polynomial invariants
The first category is that of the competition between failure modes, such as kink bands, fracture etc. Probably more of the 12 approaches fall under this very broad category than under both of the other two. The second category envisions failure at the individual fiber and matrix levels and then builds up from there. The last category, polynomial invariants, sounds mathematical but it is no more so than the other methods. The name follows from the deduction of the forms appropriate for transverse isotropy at the lamina level. Two of the failure theories are of that source. All 3 of these categories are legitimate and well motivated approaches to the failure problem for fiber composites. But each of the 12 approaches is vastly different in its individual treatment.
However, some serious reservations must be recognized and added. Most of the 12 approaches in the above 3 categories are parameters intensive. The top ranked 4 theories in both the quantitative assessment and the quant. & qual. assessment are the same 4 theories. Both that group and the final top 4 theories group (Pinho, Carrere, Puck, Cuntze) contain the following numbers of parameters (input data) in ascending order:
- 50 parameters
- 60
- 70
- 75
Not without justification, these approaches could be characterized as the power of parameters. With enough parameters just about any set of failure data could be modeled. So in this connection there is cause for concern and caution. Does it make sense that 60 or 70 or more input parameters are said to be required to model fiber composites failure while there is a credible failure theory for isotropic materials (an enormously broader class of materials) that only requires 2 parameters (strength properties) to calibrate it. Going even further, the Mises criterion is a 1 parameter form for all ductile metals. One can only imagine how many parameters would be argued to be necessary for ductile metals if the understanding of the Mises criterion did not already exist. The many parameters approach typically becomes input for black box software programs of unknown but dubious physical content.
In their conclusion the organizers expressed a strong preference and recommendation for the failure modes approach (persuasively called the physically based approach in the failure exercise and consistent with their top 4 theories). This failure modes approach is the most parameters intensive of the various methods, as discussed above. The assessment in and of this review is that there is far too little definitive and non-conflicting information in the failure exercise to make such a judgment. None of the theories as described and used in the failure exercise are even close to the maturity level needed to support any such conclusion. Actually all 12 of the approaches are in various ways physically based, but that is not enough to assure success, it’s only the price of admission.
The data sets of the failure exercise that were said to validate some models and disprove or at least disqualify others had no stated error bands. In all fiber composites assessments, the quality of the data is the matter of the first importance. It is not difficult to find examples of well regarded data that differ by as much as 50% or 100% or even more. Great differences in failure data values for composites is the rule, not the exception. That makes it all the more important that there should be at least two independent sources for all specific data sets used in all critical evaluations of failure theories. It does appear that the organizers made a genuine effort to procure meaningful data, but the realities of the field require far greater emphasis on obtaining wholly reliable data for evaluation purposes than seemed to be possible here. Fewer data cases that are carefully screened to be of much greater value and be of pivotal significance could possibly be a realistic requirement for moving forward.
Looking Ahead
Since the data based evaluation of fiber composites failure theories is so difficult, at least it would have been helpful if the failure exercise contained an independent assessment of the relative soundness of the various failure theories. This could have been a potentially helpful result. Although there were descriptions of the various approaches there were no hard core comparative theoretical evaluations. It remains for others to organize a careful, thorough and deep evaluation of the theoretical bases for the many different models of failure.
In many respects (enumerated herein) the WWFE-II assessment of failure theories constitutes an example of how an evaluation program should not be done. If so, how then should one be done? That is a question easily posed but not easily answered. Among other things the following barriers must be realistically dealt with: (i) the extreme difficulty in designing and performing multi-dimensional experiments with highly anisotropic fiber composites, (ii) the very large variability in materials samples and the materials spatial inhomogeneity with consequent uncertainty in testing results, and (iii) the obstacles to theoretical development and the methods to surmount those. Perhaps future investigators will find a reasoned and balanced answer to the basic evaluation question and to the unavoidable variability’s and uncertainties that attend all matters of fiber composites failure.
The daunting complexity of the fiber composites failure problem is undeniable. Still there is reasonable cause for optimism. The failure exercise puts a tighter focus on the problem. Avenues to be avoided are becoming more apparent through the investigation. Even more important, promising directions for major effort are becoming ever more clear. Someday there likely will be a robust and reliable failure criterion for fiber composite materials. Despite its many shortcomings, the WWFE-II provided a useful step toward that goal.
Reference
1. Kaddour, A. S., and Hinton, M. J. (2013), “Maturity of 3D Failure Criteria for Fibre-Reinforced Composites: Comparison Between Theories and Experiments: Part B of WWFE-II,” J. Comp. Mats., 47, 925-966.



